What are Complex Numbers? Complex function, Complex Number Applications

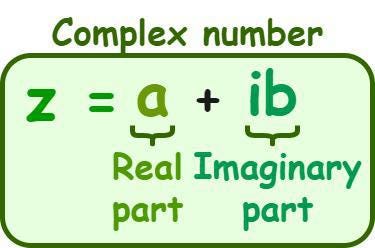
Complex analysis is a branch of mathematics that deals with complex numbers and their functions. It is a powerful tool that has many applications in physics, engineering, and other fields. The study of complex analysis involves the study of complex functions and their derivatives, as well as the study of complex numbers and their properties.

Introduction to Complex Numbers

A complex number is a number of the form a + bi, where a and b are real numbers and i is the imaginary unit. The real part of a complex number is a, and the imaginary part is b. Complex numbers can be added, subtracted, multiplied, and divided like real numbers. They can also be plotted on a complex plane, where the real part of the number is plotted on the x-axis and the imaginary part is plotted on the y-axis.

Complex Functions

A complex function is a function that maps a complex number to another complex number. Complex functions are used to model many physical phenomena, and they can be used to describe the behavior of electrical circuits, fluid dynamics, and other systems. Complex functions can be represented in a variety of ways, including power series, Laurent series, and complex integrals.

Derivatives and Analytic Functions

The derivative of a complex function is an important concept in complex analysis. A complex function is said to be analytic at a point if it is differentiable at that point. Analytic functions have many properties that make them useful for modeling physical systems. For example, analytic functions are always locally invertible, which means that they can be used to describe reversible processes.

Applications of Complex Analysis

Complex analysis has many applications in a variety of fields. For example, it is used in the study of electrical circuits, fluid dynamics, and other systems. Complex analysis can be used to model the behavior of these systems and to design new systems that are more efficient and effective. Complex analysis is also used in the field of number theory, where it is used to study the distribution of prime numbers and other number-theoretic concepts.

Conclusion

Complex analysis is a branch of mathematics that deals with complex numbers and their functions. It is a powerful tool that has many applications in physics, engineering, and other fields. The study of complex analysis involves the study of complex functions and their derivatives, as well as the study of complex numbers and their properties. Complex analysis is a fascinating field that continues to play an important role in the advancement of science and technology.